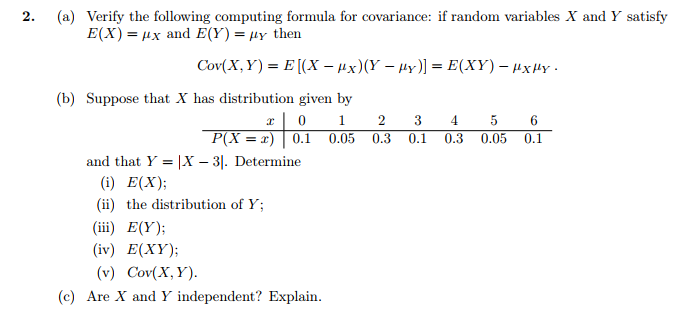
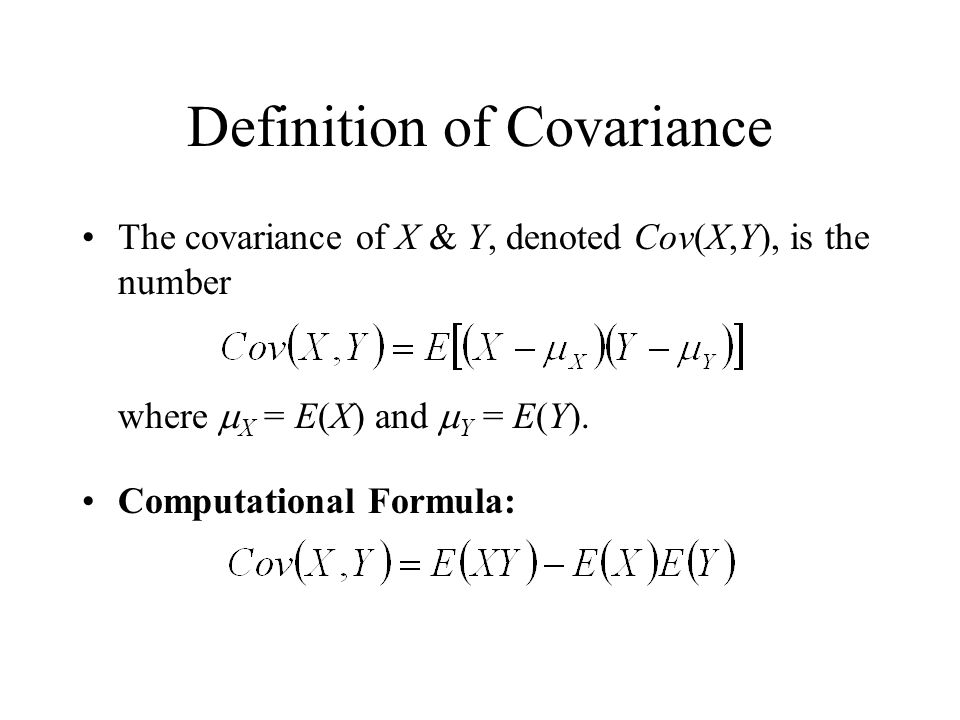
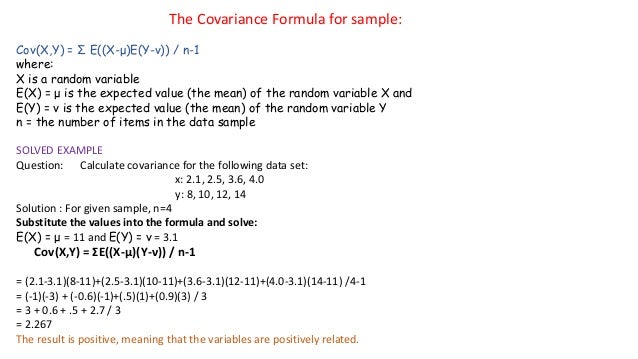
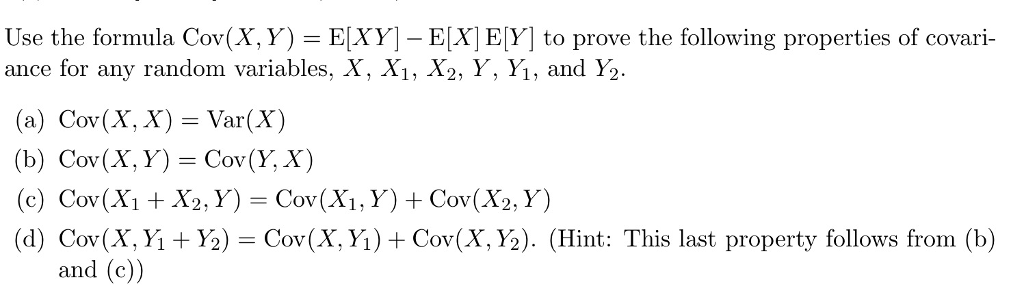Covariance Formula E Xy

ρ x y the correlation between the variables x and y.

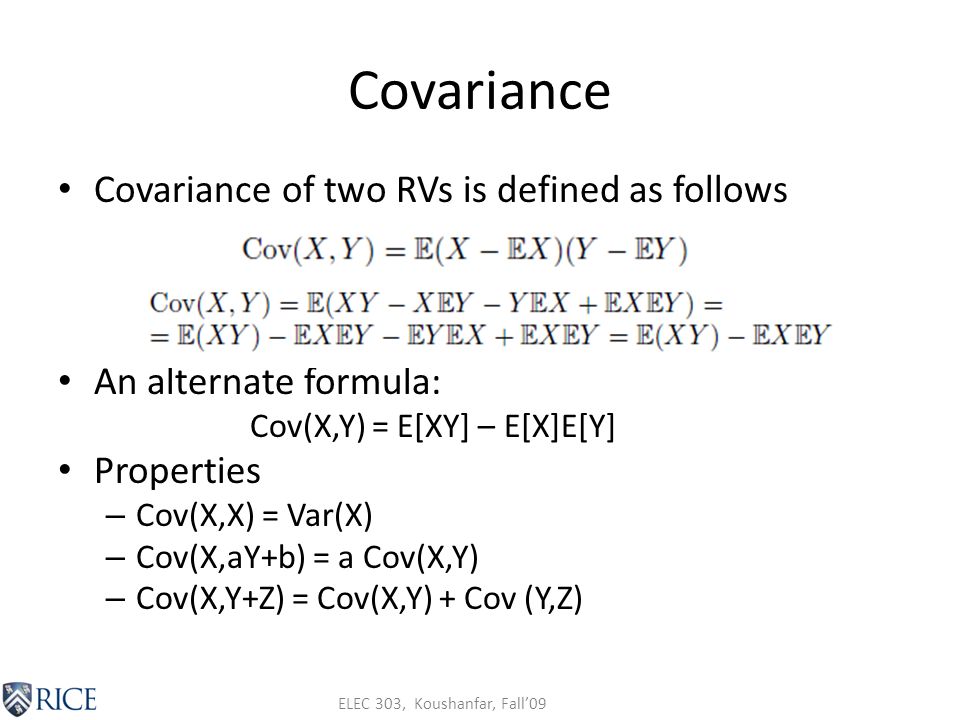
Covariance formula e xy. There is an identity for covariance cov x e xy 2 x y here s the proof. Cov x y 2 52 4. Covariance measures the degree of dependence between two random variables. ȳ mean of y.

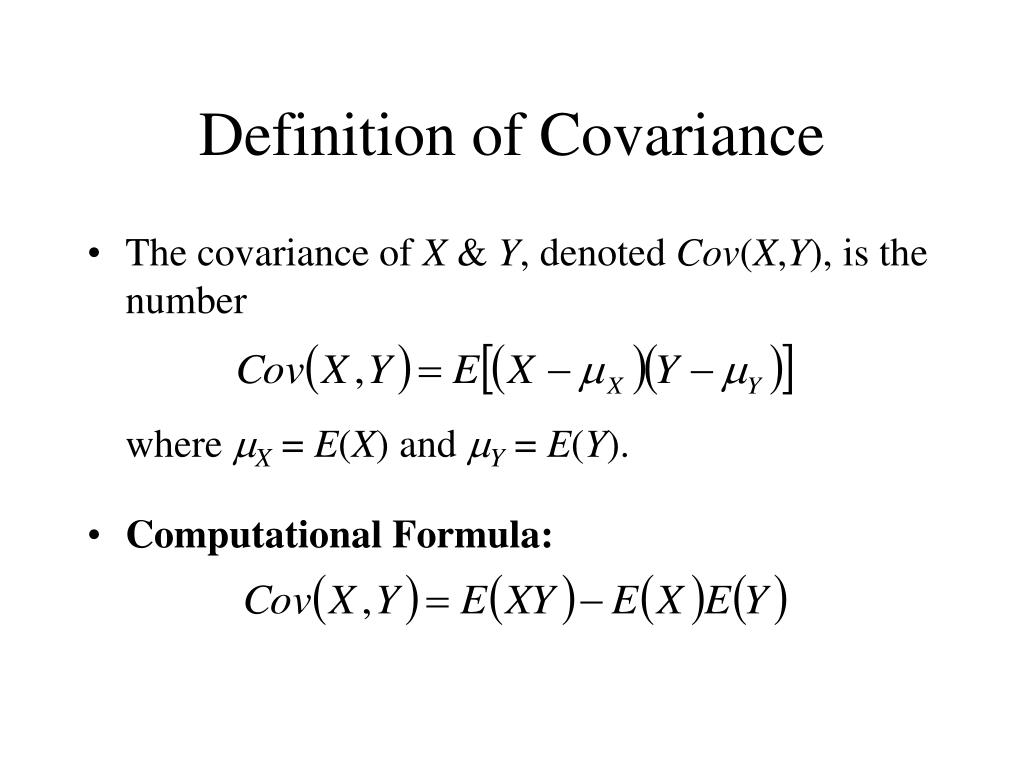
The covariance is also sometimes denoted σ x y displaystyle sigma xy or σ x y displaystyle sigma x y in analogy to variance. Y i data value of y. The covariance of two random variables x and y is defined as follows. Cov x y 0 204 0 078 0 49 0 464 1 848 4.

Eq 1 where e x displaystyle operatorname e x is the expected value of x displaystyle x also known as the mean of x displaystyle x. The outcome is positive which shows that the two stocks will move together in a positive direction or we can say that if abc stock is booming than xyz is also has a high return. John is an. X i data value of x.

The relationship between the two concepts can be expressed using the formula below. By using the linearity property of expectations this can be simplified to the expected value. Notations in the formula for covariance. It s either a positive or negative number often denoted by cov x y the large or smaller values of both x y variables result the positive score of covariance while the larger values of variable x and smaller values of variable y results the negative score.

I was trying to use e xy e x e y the second stack exchange network stack exchange network consists of 177 q a communities including stack overflow the largest most trusted online community for developers to learn share their knowledge and build their careers. N number of data values. Key takeaways covariance in finance covariance is known to be a statistical tool that can be used to determine the relationship between the movement of any two asset prices. Covariance formula example 2.

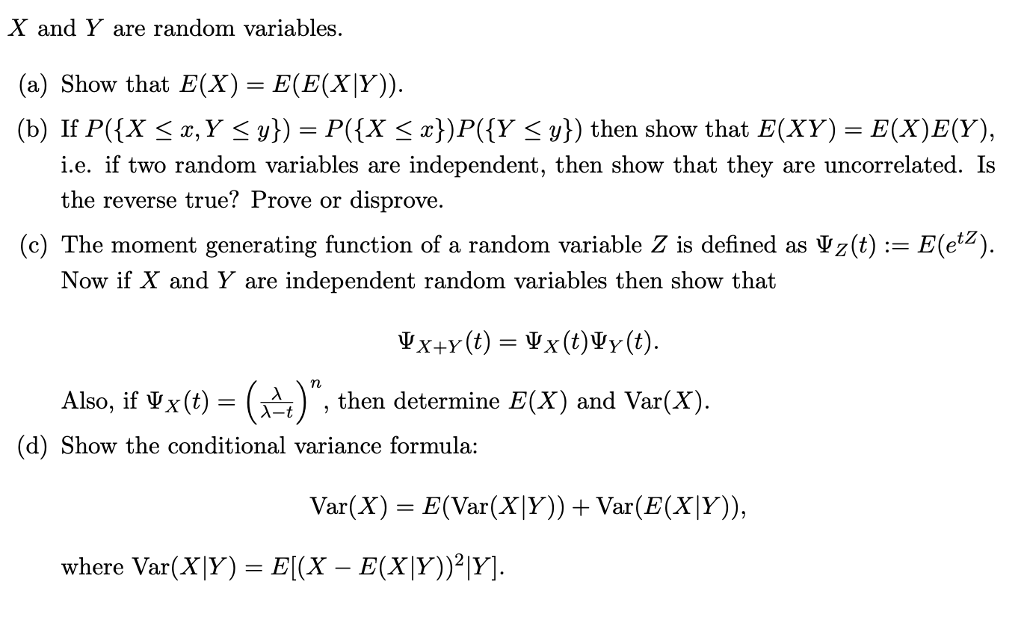
σ x the standard deviation of the x variable. Cov x y the covariance between the variables x and y. Cov x y e xy mu x mu y proof in order to prove this theorem we ll need to use the fact which you are asked to prove in your homework that even in the bivariate situation expectation is still a linear or distributive operator. Cov x y e xy e x e y.

Covariance is a method to estimate the nature of association between two random variables x y in probability statistics experiments. The covariance of the two stock is 0 63. Cov x y e x x y y e xy xy x y x y e xy xe y e x y x y e xy x y covariance can be positive zero or negative.